Understanding the Equation of a Line: y=mx+b
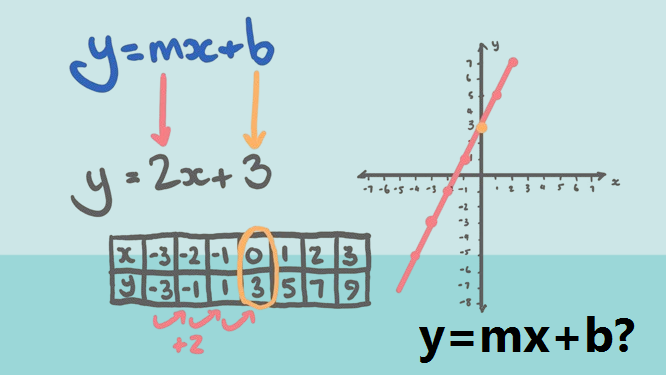
y=mx+b When it comes to understanding linear equations, the formula y=mx+b is fundamental. It’s the bread and butter of algebra, providing a straightforward way to describe a straight line. This equation might look simple at first glance, but it carries a lot of information about the relationship between two variables. In this article, we’ll break down the components of this equation, explore how it’s used, and dive into why it’s so important in mathematics and beyond.
The Basics: What Do y, m, x, and b Stand For?
Understanding ‘y’ and ‘x’
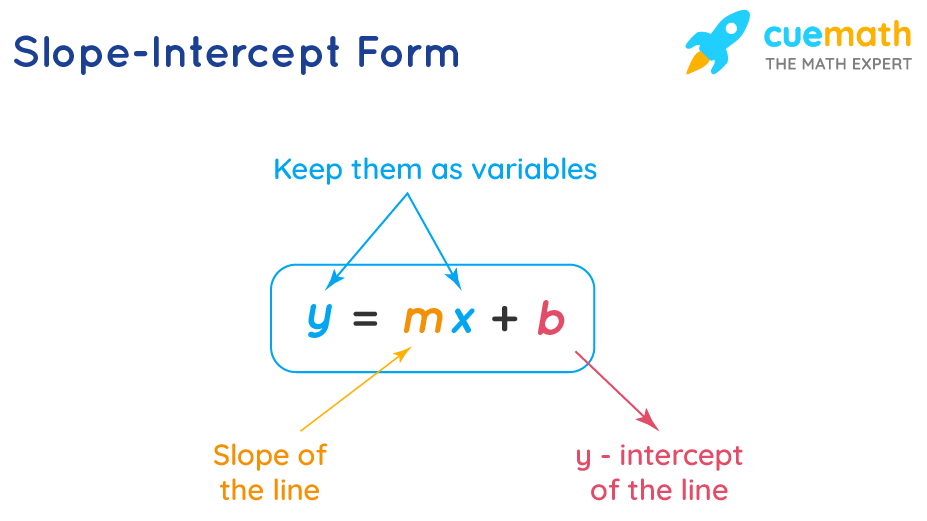
In the equation y=mx+b, ‘y’ and ‘x’ are variables. These variables represent the coordinates of any point on the line. Specifically, ‘x’ is the independent variable, which means you can choose any value for ‘x,’ and ‘y’ is the dependent variable, meaning its value depends on what you choose for ‘x.’
For example, if you set x to 1, you can use the equation to find the corresponding value of y. This relationship between x and y is the essence of what makes this equation powerful – it allows you to predict one value based on the other.
The Slope ‘m’
The ‘m’ in the equation represents the slope of the line. The slope tells us how steep the line is and the direction it goes. A positive slope means the line is rising as it moves from left to right, while a negative slope means the line is falling. The slope is calculated as the ratio of the rise (the change in y) over the run (the change in x).
For instance, if you have a slope of 2, it means for every unit increase in x, y increases by 2 units. Understanding the slope is crucial because it gives insight into the rate of change between the variables.
The Y-Intercept ‘b’
The ‘b’ in the equation is the y-intercept. This is the point where the line crosses the y-axis. When x is zero, y equals b. The y-intercept provides a starting point for the line on the graph. It tells you the value of y when x is zero, which can be particularly useful in various applications, from economics to physics.
For example, if the y-intercept is 5, this means that when x is zero, y is 5. This starting point helps to anchor the line on the graph, making it easier to plot and understand.
Plotting the Line: From Equation to Graph
Setting Up the Graph
To plot the line represented by y=mx+b, you first need to set up a graph with an x-axis and a y-axis. Label each axis and decide on a scale. It’s essential to choose a scale that allows you to accurately plot the points you’ll calculate from the equation.
Finding Points
The next step is to find points on the line. Start by choosing a value for x and use the equation to find the corresponding y value. Plot this point on the graph. Repeat this process with different values of x to get a few points. Typically, you’ll want at least two points, but more points can help ensure accuracy.
For instance, if your equation is y = 2x + 3, you can start with x = 0. Plugging this into the equation gives y = 3. So, one point is (0, 3). Next, try x = 1. Plugging this in gives y = 5, so another point is (1, 5). Continue this process to get a few points, then draw a line through them.
Drawing the Line
Once you have your points plotted, draw a line through them. Make sure the line extends across the entire graph to show the relationship between x and y clearly. This line represents all the solutions to the equation y=mx+b. Every point on this line is a solution to the equation, meaning if you plug in the x and y coordinates of any point on the line into the equation, it will hold true.
Applications of y=mx+b in Real Life
Economics and Business
In economics and business, the equation y=mx+b is used to model relationships between different variables. For example, in a simple linear cost model, y could represent the total cost, x could be the number of units produced, m could be the variable cost per unit, and b could be the fixed cost.
This model helps businesses predict their total costs based on the number of units they plan to produce. By understanding the slope and y-intercept, businesses can make informed decisions about production levels, pricing, and profitability.
Physics and Engineering
In physics and engineering, this equation is used to describe motion and forces. For instance, in kinematics, the position of an object moving at a constant velocity can be described by the equation y=mx+b, where y is the position, m is the velocity, x is time, and b is the initial position.
This simple linear model is a powerful tool for predicting future positions of moving objects. It’s fundamental in designing systems and understanding physical phenomena.
Everyday Life
Even in everyday life, you can see the principles of y=mx+b in action. For example, consider the relationship between time spent studying and test scores. If there’s a linear relationship, you could use this equation to predict test scores based on study time.
This relationship helps students understand the importance of their study habits and how they directly impact their performance. By recognizing and applying this equation, students can better manage their time and improve their results.
Common Misconceptions and Challenges
Misunderstanding the Slope
One common misconception is misunderstanding what the slope represents. Some might think a slope of 2 means you go up 2 units for every 2 units you go over, but actually, it means you go up 2 units for every 1 unit you go over. Understanding the correct interpretation of the slope is crucial for accurately using the equation.
Confusing the Y-Intercept
Another challenge is confusing the y-intercept. It’s easy to mix up the y-intercept with other points on the graph. Remember, the y-intercept is specifically where the line crosses the y-axis, which happens when x is zero. Keeping this in mind helps in correctly plotting and interpreting the graph.
Graphing Mistakes
Graphing errors are also common, especially if the scale is not chosen properly or points are plotted inaccurately. Ensuring that your graph is correctly scaled and your points are accurately plotted is essential for a clear and correct representation of the equation.
Conclusion: The Power of y=mx+b?
The equation y=mx+b is more than just a formula; it’s a powerful tool that helps us understand and predict relationships between variables. Whether in business, science, or everyday life, this equation provides a clear and straightforward way to model and analyze linear relationships.
y=mx+b By breaking down the components of the equation, understanding how to plot it on a graph, and recognizing its real-life applications, we gain valuable insights into the world around us. Despite its simplicity, y=mx+b opens the door to deeper mathematical concepts and practical problem-solving skills that are essential in various fields.